I will…
- gain an intuition about the ostensibly unfathomable quantum domain
- get acquainted with probability distributions and randomness
- implement my own ideas on high-performance computers
Summary:
In contrast to the Newtonian orbits of two planets, the trajectories of three display chaotic behaviour. This discovery of a strong sensitivity of the temporal evolution of a system whose dynamics rests on the same interactions as one of its subsystems, stripped of one object, on initial conditions has its analogue in the quantum world.
While studying the 3-hydrogen nucleus, L.H. Thomas discovered that one can describe the 2-hydrogen subsystem (cf. the stable Kepler orbits) with a central force between the neutron and proton of arbitrarily short range. When he employed this interaction if an neutron is added to the neutron-proton system, he could predict that the ensuing solutions are unstable (cf. “chaos”).
Given the insight provided by the visualization of classical orbits and the intuition gained by plots of probability densities of an electron in the hydrogen atom, this projects aims to visualize Thomas-collapsing 3-body wave functions.
Outline:
- How does one visualize probability densities in quantum mechanics in general?
- How would I visualize not a 1-body, but a 2-body wave function describing the relative motions within the 3-body system?
- What is the precise mathematical form of the wave function to plot?
- How do I runtime optimize my simulation?
Solution (Shoaib Ahmed Mohammed, BSc, SRM AP):
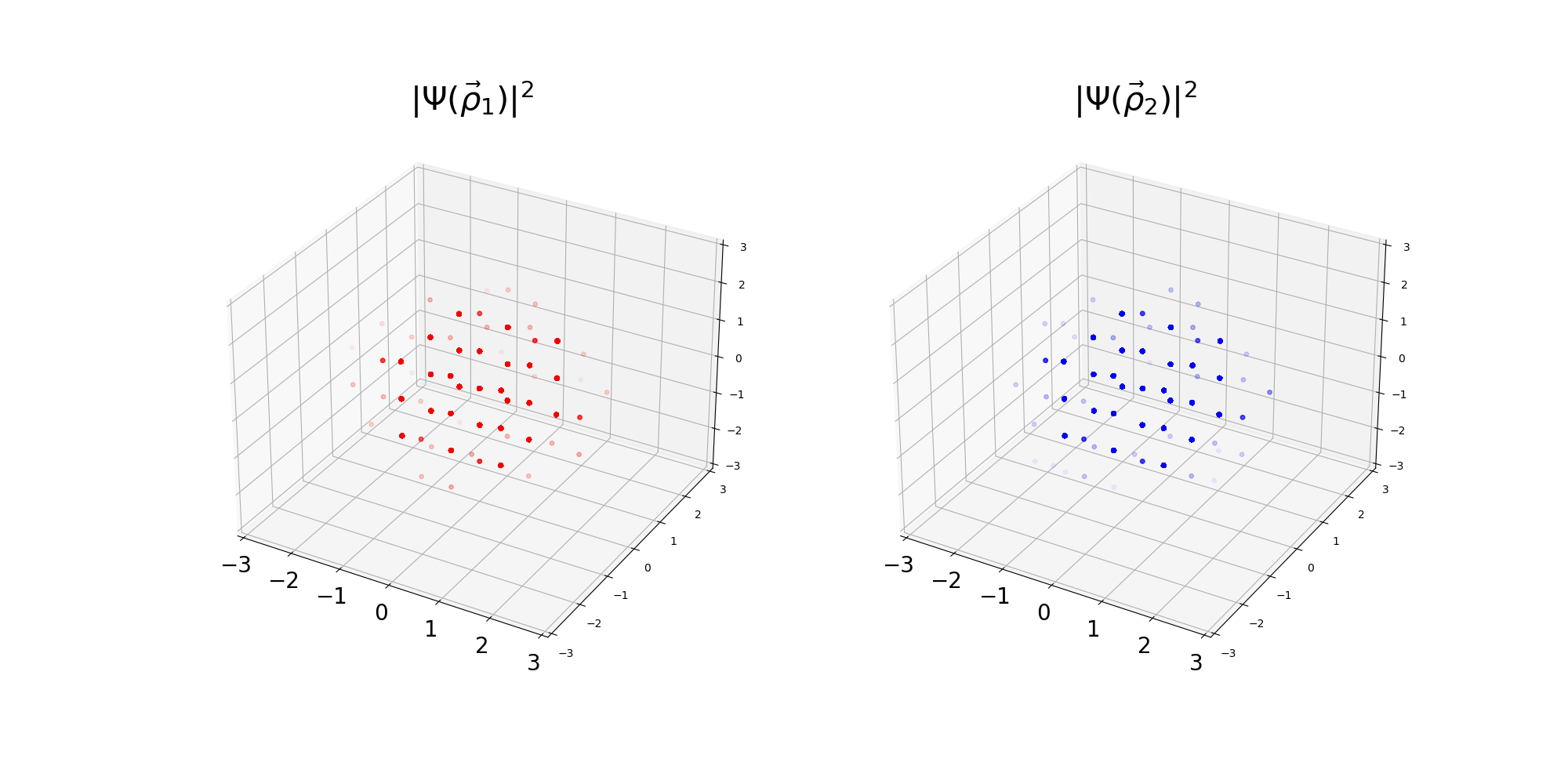
Resources:
- Thomas’ original work on the collapse of the 3-body wave function in the context of nuclear physics
- Efimov’s discovery of universal 3-body quantum behaviour
- Anderson’s essay on the emergence of complexity and the refutation of a reductionist approach to science